Law of Constant Returns/Law
of Constant Cost:
(Version of Classical and Neo Classical
Economists):
Definition and Explanation:
The law of constant returns
also called law of constant cost. It is said to operate when with the
addition of successive units of one factor to fixed amount of
other factors, there arises a proportionate increase in total
output. The yield of equal return on the successive doses of
inputs may occur for a very short period in the process of
production. The law of constant return may prevail in those
industries which represent a combination of manufacturing as
well as extractive industries.
On the side of manufacturing
industries, every increased investment of labor and
capital may result in a more than proportionate increase in the
total output. While on the other extractive side, an increase in
investment may cause, in general, a less than proportionate
increase in the amount of produce raised. If the tendency of the
marginal return to increase is just balanced by the tendency of
the marginal return to diminish yielding an equal return, we
have the operation of the law of constant returns. In the words
of Marshall:
"If the actions of the law of increasing and
diminishing returns are balanced, we have the law of constant
return".
In actual life, the law of constant
returns can operate only if the following conditions are
fulfilled:
(i) There should not be any increase
in the prices of raw materials in the industry. This can only be
possible if commodities are available in large supply.
(ii) The prices of various factors
of production should remain the same. The .supply of various
factors of production needed for a particular industry should be
perfectly elastic.
(iii) The productive services should
not be fixed and indivisible.
If we study the above mentioned
conditions carefully, we will easily conclude that in the actual
world, it is not possible to find an industry which obeys the
law of constant returns. The law of constant returns can operate
for a very short period when the marginal return moves towards
the optimum point and begins to decline. If the marginal return,
at the optimum level remains the same with the increased
application of inputs for a short while, then we have the
operation of law of constant returns. The law is represented now in the form of a table and a
curve.
Schedule:
|
Productive doses
|
Total
Return (meters of cloth) |
Marginal
Return (meters of cloth) |
|
1 |
60 |
60 |
|
2 |
120 |
60 |
|
3 |
180 |
60 |
|
4 |
240 |
60 |
|
5 |
300 |
60 |
In the table given above, the
marginal return remains the same, i.e. 60 meters of cloth with
the increased investment of inputs.
Diagram/Graph:
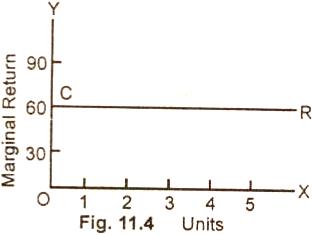
In figure (11.4) along OX are
measured the productive resources and along OY is represented
the marginal return. CR is the fine representing the law of
constant returns. It is parallel to the base axis.
Relevant Articles:
|